When heating up ice, the first thing we need to notice is that the curve is not a straight line, but has 5 straight segments. Each segment is " ruled " by a different equation. To calculate the total energy change (delta H) of a change, calculate the energy of each segment and add them together.

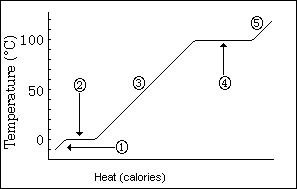
1. In this region we are heating ice. The formula for how energy is needed is: ΔH = m * ΔT*Cp.  

Where ΔH   is the change in energy needed, m  is the mass of ice being warmed or cooled, ΔT  is the temperature change (Ending T- Beginning T), and Cp  is the specific heat of the ice (0.5 cal/g °C)

2. In this region a curious thing happens, the temperature neither goes up or down, all the heat energy is used in melting or freezing. The formula for how energy is needed is:  ΔH = m * Hf

Where ΔH   is the change in energy needed, m  is the mass of ice melted or the mass of water being frozen, Hf is the heat of fusion of ice (80 cal/g)

3. In this region we are warming water, with no phase change so the equation is: ΔH = m * ΔT*Cp.   

Where ΔH   is the change in energy needed, m  is the mass of ice being warmed or cooled, ΔT  is the temperature change (Ending T- Beginning T), and Cp  is the specific heat of the water (1.0 cal/g °C)

4. In this region another phase change occurs.Water is converted to steam (or steam to water) without any temperature rise.  The formula for how energy is needed is:  ΔH = m * Hv

Where ΔH   is the change in energy needed, m  is the mass of water vaporized or the mass of steam being condensed, Hv is the heat of vaporization of water (a huge 540 cal/g)

5. In this region we are heating steam. The formula for how energy is needed is: ΔH = m * ΔT*Cp.  

Where ΔH   is the change in energy needed, m  is the mass of steam being warmed or cooled, ΔT  is the temperature change (Ending T- Beginning T), and Cp  is the specific heat of the steam (0.5 cal/g °C)

To solve numerical problems plot the beginning point and ending point on the graph and break the heating into small segments (up to 5) calculate the energy change for each step and add them together for the total energy change of the total "trip". Calculate using only positive numbers and assign a sign at the very end: left and down represents - calories; up and right is + calories.

In Mr. K's class we will use calories so we can relate to food calories (kilocalories), and to relate to the rest of the world we will use joules (pronounced jewels).

1 calorie [thermochemical] = 4.184 joule  1 food Calorie = 1000 chemistry calories = 1 kcal.

Note: physicists (and some chemists) use Q to denote energy. If we only work at constant pressure (usually atmospheric) then Q = ΔH. Their terminology is a little more universal it allows for PdV work. Ours is a little more simple and ΔH reminds me of heat.