
Chapter 4:
Electrolytes:
Electrolytes conduct electricity when dissolved because they produce ions in aqueous solutions
Strong Electrolytes: lots of ions
Weak Electrolytes: few ions
Non-electrolytes: molecular substance
Non-ionic. Covalently bonded molecular substance
Non-metals
Acids:
Strong acid = strong electrolyte
Ion that acids make is H+ (protons)
Weak acids = weak electrolyte
Mostly stay together as molecules
Few H+ ions produced
Precipitation reactions
Double Displacement Reactions leading to insoluble product.
Start using states of matter in equations
(aq) dissolved, (s) solid, (l) liquid, (g) gas
Memorize or at least very familiar with solubility rules.
Displacement Reactions
DDR's often Redox reactions
AB + CD ==> AD + CB
SDR's always Redox reactions
A + BC ==> AC + B if metal
A + BC ==> AB + C if non-metal
Net Ionic Equations
This is a big change from Reg Chem. All equations that can be put in Net Ionic format MUST be put in Net Ionic format.
Write complete balanced equation, with states of matter
Rewrite Equation
Ionize, dissociate anything soluble
Cancel spectator ions.
Write net ionic equation
Acid/Base Reactions
Learn Strong Acids: If not strong must be weak
Learn Strong Bases: Group I and Soluble Group II hydroxides
Weak base: ammonium hydroxide, amines.
Neutralization: Acid + Base ==> Salt + Water
Net ionic equation is always H+(aq) + OH-(aq)==> H2O(l)
Exception: if base is insoluble it is not present as ions so can't cancel spectators
Redox (Review your notes from Reg. Chem.)
Chapter 5 Thermodynamics
Energy and work and heat are equivalent
First Law of Thermodynamics Energy is conserved
Exothermic reactions release stored heat to environment
Delta H system is -
Delta H environment is +
Enthalpy is state function (stored heat)
Enthalpies of Reaction (both physical changes and chemical changes)
kJ or kJ/mol Check to see if the reaction produces many moles or just 1
A reaction that burns 4 moles of propane will produce more energy than one that burns 2 moles.
CH4 (g) + 2 O2 (g) --> CO2 (g) + 2 H2O (g) deltaH = -802 kJ ( per mole of CH4 implied)
2CH4 (g) + 4 O2 (g) --> 2CO2 (g) + 4 H2O (g) deltaH = -1604 kJ ( per 2mole of CH4 implied)
Example: How many joules are released by the burning of 28.0 grams methane in air, given that delta Hcombustion methane is -802 kJ/mol
Balance equation: CH4 (g) + 2 O2 (g) --> CO2 (g) + 2 H2O (g) deltaH = -802 Kj/mol
Factor label style:
| 28.0 g methane | 1 mole methane | -802 kJ |
| 16.06 g methane | 1 mole methane |
= 1298.3 kJ
Hess' Law. Since Enthalpy is a state function it is independent of path. So we can add subtract or multiply intermediate reactions and if we can get them to add up to the equation of interest we can calculate the overall enthalpy change by doing the same algebraic step to the enthalpies of reaction. Flip the sign if the equation is reversed, multiply by 3 if reaction tripled etc.
Example: Calculate the enthalpy of reaction for: 2S (s) + 3O2 (g) --> 2 SO3 (g)
Given:1.) S (s) + O2 (g) --> SO2(g) deltaH= -296.9kJ
2.) 2SO3(g) --> 2SO2(g) + O2(g) + 196.6 kJ
Want 2S as a reactant, multiply reaction #1 by 2 remember multiply enthalpy also
2S (s) + 2O2 (g) --> 2SO2(g)
deltaH= (2)-296.9kJ
2SO2(g) + O2(g) -->
2SO3(g)
(-1) 196.6 kJ
2S (s) + 3O2 (g) --> 2 SO3 (g) -790.4 kJ
Enthalpies of formation
Enthalpy required to make 1 mole of production from its constituent elements in their stable form at 25 °C and 1 atm. Units are kJ (per 1 mole is implied by definition)
Enthalpy of formation of any element in its stable form at 25 ºC and 1 atm is 0 kJ.
Calorimetry
Key concept: Heat lost by the hot object's cooling will be gained by the cool object's warming.
Heat capacity: J/gºC how much energy is required to raise the temperature of a substance.
Calorimeter constant J/°C how many Joules it takes to change a calorimeter's temperature 1°C
(Must keep the calorimeter parameters constant: use exact same mass of water each trial.
Heat capacity of water 4.184 J/gºC
Heat of fusion of water 333.5 J/g energy required to melt (+) or liberated when freezing water.
Heat of vaporization of water 2257 J/g (+) vaporizing, (-) condensing.
Key relationship deltaH = m Cp delta T
Example easy:
25.0 grams of water at 0 c is added to a calorimeter at 22.5 C if the ending temperature is 16 C What is the calorimeter constant.
Heat gained by cold object is lost by warm object or reaction
Heat gained by cold
25.0 grams x 4.184 J/g ºC x (16 ºC - 0 ºC) = + 1673.6 J.
Calorimeter constant is Energy per degree of temp change
-1673.6J/ -6.5 Cº = 257.5 J/ºC
Now running a reaction in the exact same calorimeter, If the calorimeter is seen to rise 21.0 ºC how many calories are released by the chemical reaction?
257.5 J/ºC x 21 ºC = 5407.5 J since gain by calorimeter = loss by chemicals of reaction
- 5407.5 J.
Example- more thorough and complicated
Modern Concept of the Atom/Atomic Structure (Chapter 6 Brown, LeMay and Others)
Chapter 6: Atomic Structure and periodicity:
Electromagnetic radiation: Is light a particle or a wave? Is matter energy?
The smaller a bit of matter, the more wave-like it becomes.
EMR: forms of light so.. c = λν where λ is wavelength and v is frequency and c is the speed of light (2.9979 x 108 m/s)
Nature of matter:
Energy is quantized. Can only be gained or lost in whole number steps. ΔE = nhv
n is quantum integer 1, 2, 3 etc. h is Planck’s constant 6.626 x –34 J∙s v =frequency of light.
Photoelectric effect
| Specific threshold frequency (not amplitude!!!! in sharp contrast to mechanical waves) |
Light below threshold frequency cannot remove electrons no matter what the intensity of the light
For light above the threshold frequency increasing intensity does increase numbers of electrons.
| Above threshold, KE electron increases linearly as v light increases. |
hv0 is the energy required to remove the electron from the metal.KE electron = ½ mv2 = hv - hv0
Where hv is the energy of the incident photon
Somewhat related
E=mc2
So
m = E/c2
but we say that photons have no rest mass.
Dual nature of Light
Creates interference patterns, reflects, refracts just like waves
Photoelectric effect best explained as particles
Which is it?
Both ???!!!!!
DeBroglie equation
λ = h/mv v is velocity
Does regular particulate matter have a ‘wavelength’ Apparently yes
Electron diffraction by NaCl crystals have verified DeBroglie’s eqn.
Hydrogen Spectra and the Bohr Model.
When hydrogen is excited by passing an electric arc through it. Only 4 visible spectra are emitted. Evidence that energy in the atom is quantized λ’s 410 nm, 434 nm, 486nm and 656. All hydrogen always the same. Only some wavelength possible implies that only some energy levels are present. So electrons are jumping between 4 orbitals or 4 n-numbers.
Bohr eqn: En= -2.178 x 10-18 J (1/n2)
ΔE = E end level – E start level
If moving from high orbital level to low orbital level, electron must lose energy, and ΔE will be negative. If moving from low to high ΔE will be positive.
Relating ΔE to wavelength: ΔE = hc/λ
h is Planck’s constant: 6.626 x 10–34 J∙s
c is speed of light: 2.9979 x 108 m/s
λ = hc/ ΔE
Quantum model of atom
- Only certain wave functions are allowed for orbiting electrons

The electrons travel in orbitals and have discrete quantized momenta, and therefore quantized energies. That is, not every orbit is possible but only certain specific ones, at certain specific distances from the nucleus. The electrons will not slowly lose energy as they travel, and hence will remain in stable, non-decaying orbits.
Other points are:
When an electron makes a jump from one orbit to another, the energy difference is carried away (or supplied) by a single quantum of light (called a photon)which has an energy equal to the energy difference between the two orbits (given by evaluating Bohr's equation)
The allowed orbits depend on quantized (discrete) values of orbital angular momentum
The lowest value of n is 1. This corresponds to a smallest possible radius of 0.0529 nm. This is known as the Bohr radius and an electron may get no closer to the proton.
These quantized wave forms define electron orbitals that have a probability
of being occupied by an electron p=0.9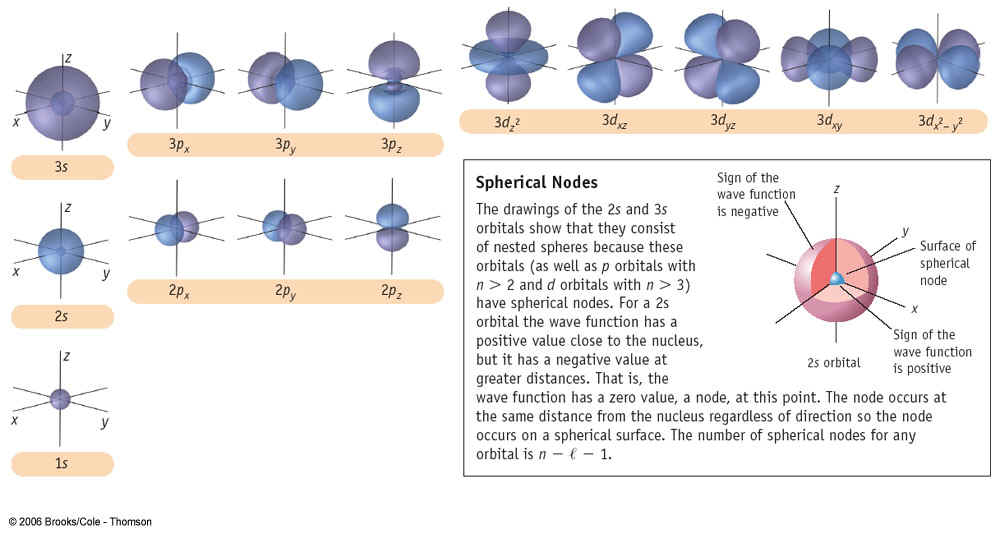
4 Quantum numbers are needed to describe each electron in a many electron atom (i.e. more complex than H)
n : Principal quantum number n= 1 to 7. Has a real world connotation of size. 2's bigger than 3's etc.
l: angular quantum number l= 1 to 3. Has a real world connotation of shape. l= 1's are similar in shape
m: magnetic quantum number m = -7 to + 7 Has a real world connotation of orientation in space.
s: quantum spin s= +1/2 or - 1/2 Electrons must be spin paired in order to share an orbital.
Period Trends: You must get this under your belt. It allows you to predict outcomes that otherwise are simply a guess.
| Characteristic | Trend (left to right) | Reason |
| Atomic radius | decreases in size from left to right | increased attractive force (acting on the same energy shell) from the nucleus as the number of protons (and hence the nuclear charge) increases |
| Ionic radius | decreases across the period until formation of the negative ions then there is a sudden increase followed by a steady decrease to the end | In general as above. The sudden increase on formation of negative ions is due to the new (larger) outer shell |
| Electronegativity | Increases | More electron attracting power of the larger nuclear charge as we move to the right |
| Metallic character | Decreases - Na, Mg, Al metals; Si metalloid; P, S, Cl, Ar non-metals | Metallic character is a measure of the ease of loss of electrons from the outer shell. This decreases with increasing nuclear charge. |
| Oxides | Na, Mg - alkaline Al - amphoteric Si, P, S, Cl -acidic |
|
| Chloride character | NaCl - ionic MgCl2 - some covalent character AlCl3 - covalent The remainder covalent |
Increasing charge density of the positive ion polarises the chloride ion as we move to the right hand side |
| Melting point | Na |
Increasing availability of electrons in the metallic bonding associated with greater charge density of the metal ion |
| Si massive increase | Si giant macromolecular structure | |
| P large decrease | P4 molecules | |
| S small increase | S8 molecules | |
| Cl |
Cl2 molecules and Ar atoms |
Electron affinity
Ionization energy
